reminded about . ()
if ugly then
1) you have three doors to choose from, there is a prize behind one of the doors.
2) you make a choice – probability of getting a prize = 1/3
3) after you have made a choice (but none of the doors are open yet) they open the door for you, behind which there is definitely no prize and ask, do you want to change your choice now.
4) it is obvious that now there are only two doors in front of you and the probability of receiving a prize is now 1/2, which is higher than 1/3. (simplifying a little, in the language of probability it will be prettier)
this whole thing is called a paradox because, what most people think, that an open door does not carry additional information, so there is no point in changing the choice. in terms of probabilities – the choice needs to be changed, because 1/2 this is better, how 1/3.
in fact, it's a paradox of a paradox.
in real conditions two choices 1 door from 3, and than 1 door from 2 – are independent. since the choice is made randomly in principle, so it is correct to run your random generator again and point to one of the two remaining doors randomly (if you point to the same, as for the first time – then you don't change the choice, if on another – then change). thus, the formation of the problem conditions leads to a composition error and we focus on formulas instead of reality;)
ie. you make two independent choices, therefore the question is “change or not change a decision” leads to an erroneous perception of reality. if anyone knows empirical experiments – share, sure, that the result will be unexpected. can it be like this: the strategy of random selection of one of the two doors will outperform the strategy of constant selection “new door”.
economists and quanta love to fall for such things. the most striking example of the discrepancy between theory and reality (of those at hand) described by William Feller flipping a coin (popularization from Mandelbrot).
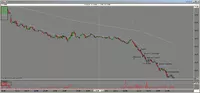
we toss a coin. if the eagle – we write +1 dollars, tail – minus dollar. tossing results are summed up. on shorts (sorry for the quality, photo by phone page from “naughty market in” Mandelbrot) the third schedule – this is the result after 10000 tossing. On the top chart – First 500, second from 500 to 5000.
as we see, if we bet on, that in the long run, our result will tend to zero (what in theory) we may face a situation where market tossing “goes against us”. this is where LTCM flew. managers believed, that the spreads will converge. and the spreads converged in the end. but these “deviations from zero” proved to be sufficient, to knock LTCM out of the saddle.
the practical conclusion is – what is risk management – is the ability to make a decision about, that it's time to change the principle of decision making. for example LTCM – fix losses having felt, that the situation is out of control.
all in all, no one is going anywhere from the need to make decisions on their own.
update.
with the independence of choice in Monty Hall, I seem to be too clever. apparently I was not able to express the idea concretely enough, so the criticism fell on, which is so clear. but, from the choice of 1 out of 2, you can go to the choice of 1 out of 3 and do the same algorithm as in Monty Hole. then the treated probability 2/3 will be higher than the initial probability 1/2 at the initial choice of 1 of them 2. ie. the math trick itself is universally applicable. although it is not known at the practical level whether the strategy of Monty-Hall will outplay the strategy of 2 equal random choices (because of, what is critical is the random distribution of the position of the prize and the door in repeated experiments).
Again, that I did not dispute and do not dispute the validity of the paradox. I thought, what is the focus in specific conditions, but he's in mathematical logic, which consists in reducing the unknown. and, the more we reduce the initial probability (for example, turning choice 1 from 2 to choose 1 from the 10th) the greater the statistical advantage we get when we change the choice.
so i was wrong,
but the fact that I was not shown an error is a consequence of the poor-quality formulation of my idea.